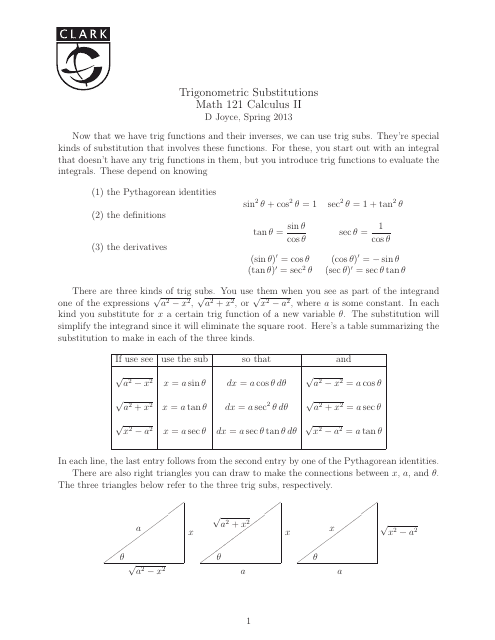
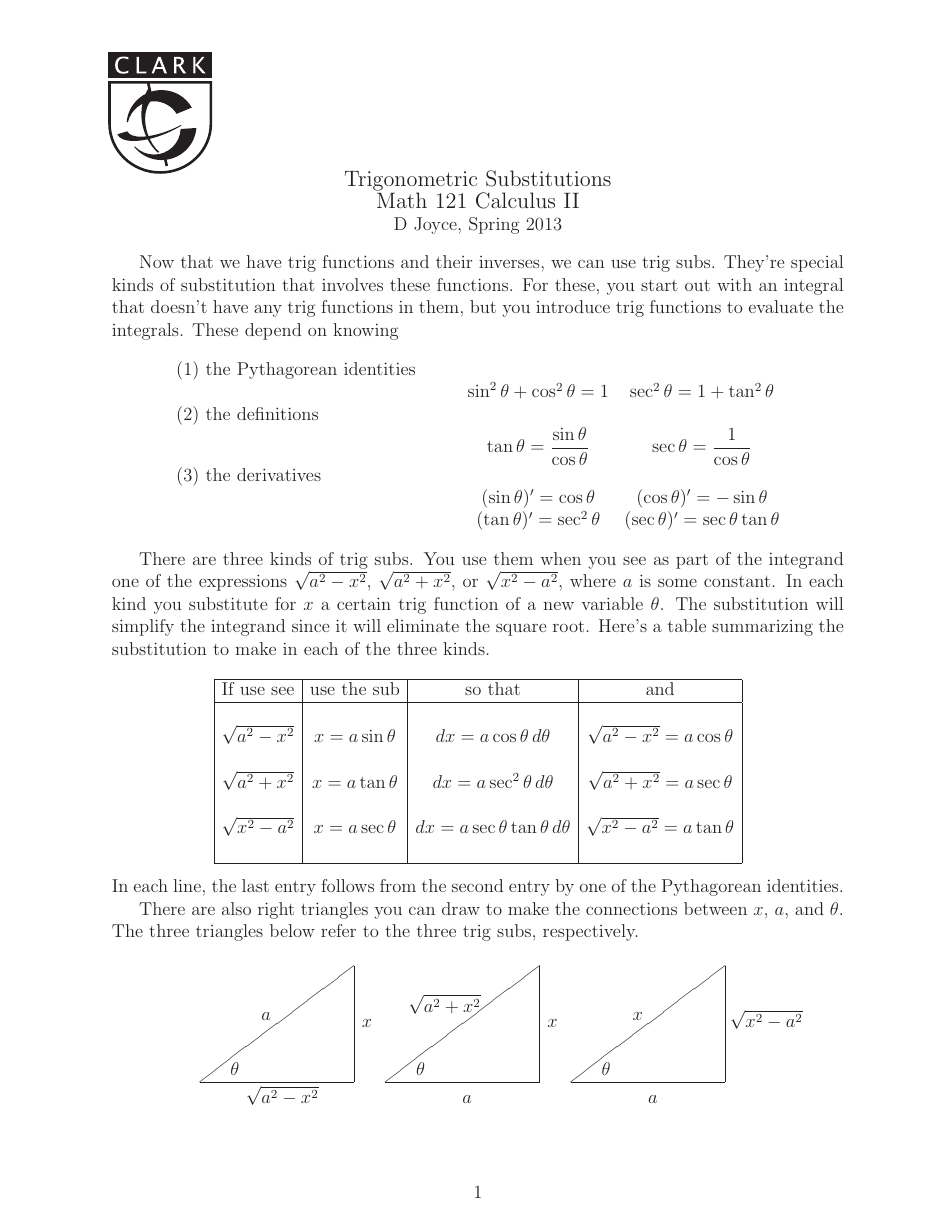
Math Calculus Cheat Sheet - Trigonometric Substitutions

A Math Calculus Cheat Sheet - Trigonometric Substitutions is a reference tool that provides formulas and techniques specifically related to using trigonometric substitutions in calculus. It helps students and learners to solve complex problems involving trigonometric functions and integrals.

FAQ

Q: What is a trigonometric substitution?
A: A trigonometric substitution is a technique used in calculus to simplify integrals that involve trigonometric functions.

Q: What are the common trigonometric substitutions?
A: The common trigonometric substitutions are: x = sin(t), x = cos(t), and x = tan(t).

Q: When should I use trigonometric substitutions?
A: Trigonometric substitutions are typically used when you have a square root of a quadratic expression in your integral.

Q: How do I choose the appropriate trigonometric substitution?
A: To choose the appropriate trigonometric substitution, look for the square root of a quadratic expression and match it with the corresponding trigonometric substitution.

Q: What is the general formula for trigonometric substitutions?
A: The general formula for trigonometric substitutions is: x = h(t), where h(t) is a trigonometric function and x is the variable of integration.

Q: What are the benefits of using trigonometric substitutions?
A: Trigonometric substitutions can help simplify integrals and make them easier to solve by introducing trigonometric identities.

Q: Are there any limitations to using trigonometric substitutions?
A: Trigonometric substitutions may not always be applicable and may not work for every type of integral.

Q: Can I use trigonometric substitutions in every calculus problem?
A: No, not every calculus problem will require the use of trigonometric substitutions. They are specific techniques used for certain types of integrals.