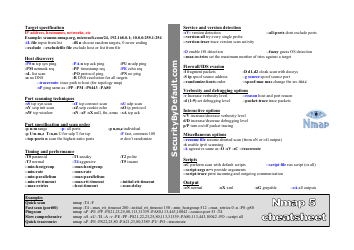
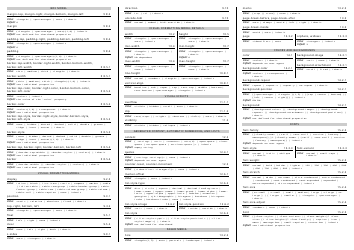
Exponents and Logarithms Cheat Sheet

The Exponents and Logarithms Cheat Sheet is a reference tool that provides quick and concise explanations and formulas related to exponents and logarithms. It can be used by students, professionals, or anyone who needs a quick reminder or guide on these mathematical concepts.

FAQ

Q: What is an exponent?
A: An exponent is a number that represents the power to which another number is raised.

Q: What is a logarithm?
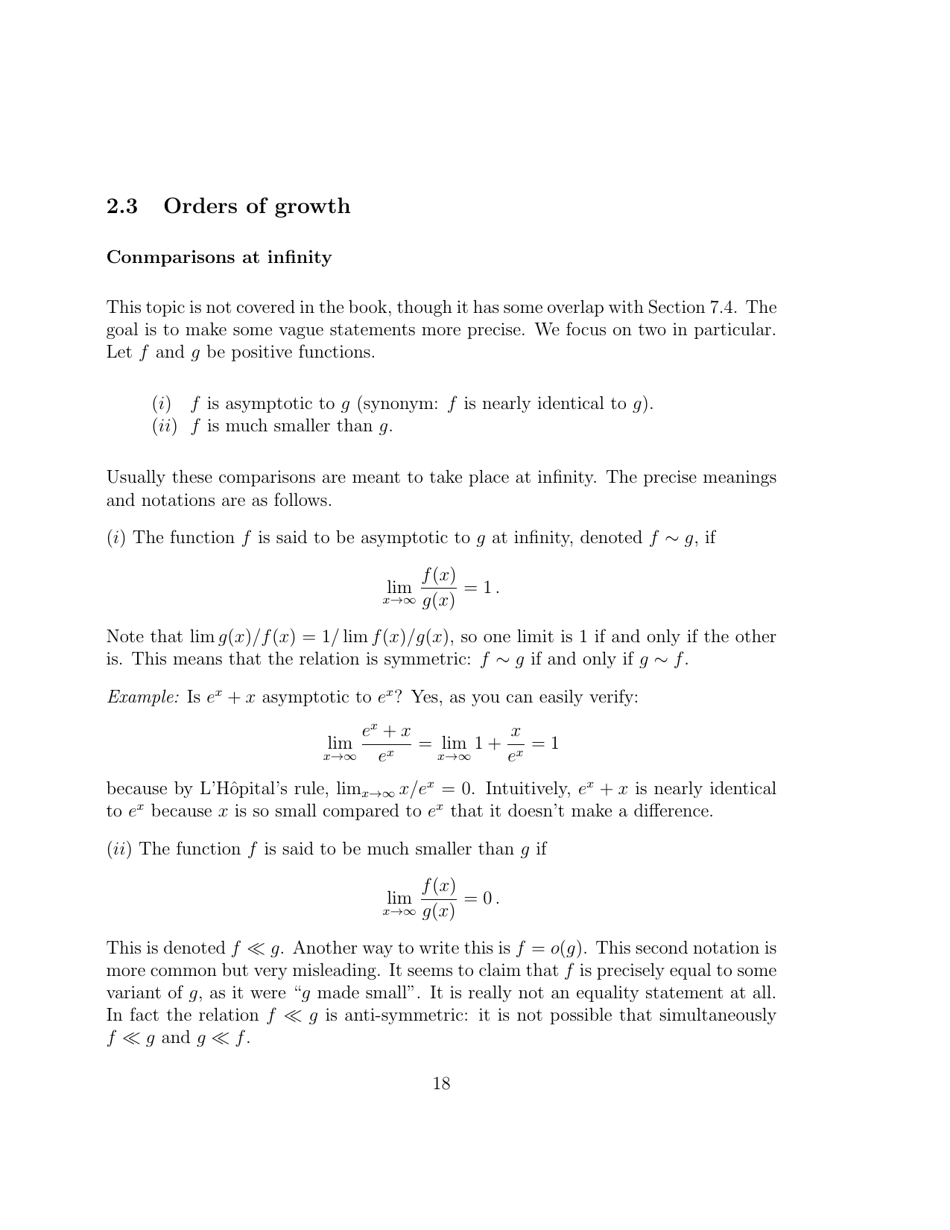
A: A logarithm is the inverse operation of exponentiation. It helps determine the exponent needed to obtain a certain number.

Q: What are the basic properties of exponents?
A: The basic properties of exponents include the product rule, quotient rule, and power rule.

Q: What is the product rule for exponents?
A: The product rule states that when multiplying two numbers with the same base, you can add their exponents.

Q: What is the quotient rule for exponents?
A: The quotient rule states that when dividing two numbers with the same base, you can subtract their exponents.

Q: What is the power rule for exponents?
A: The power rule states that when raising a number with an exponent to another exponent, you can multiply the exponents.

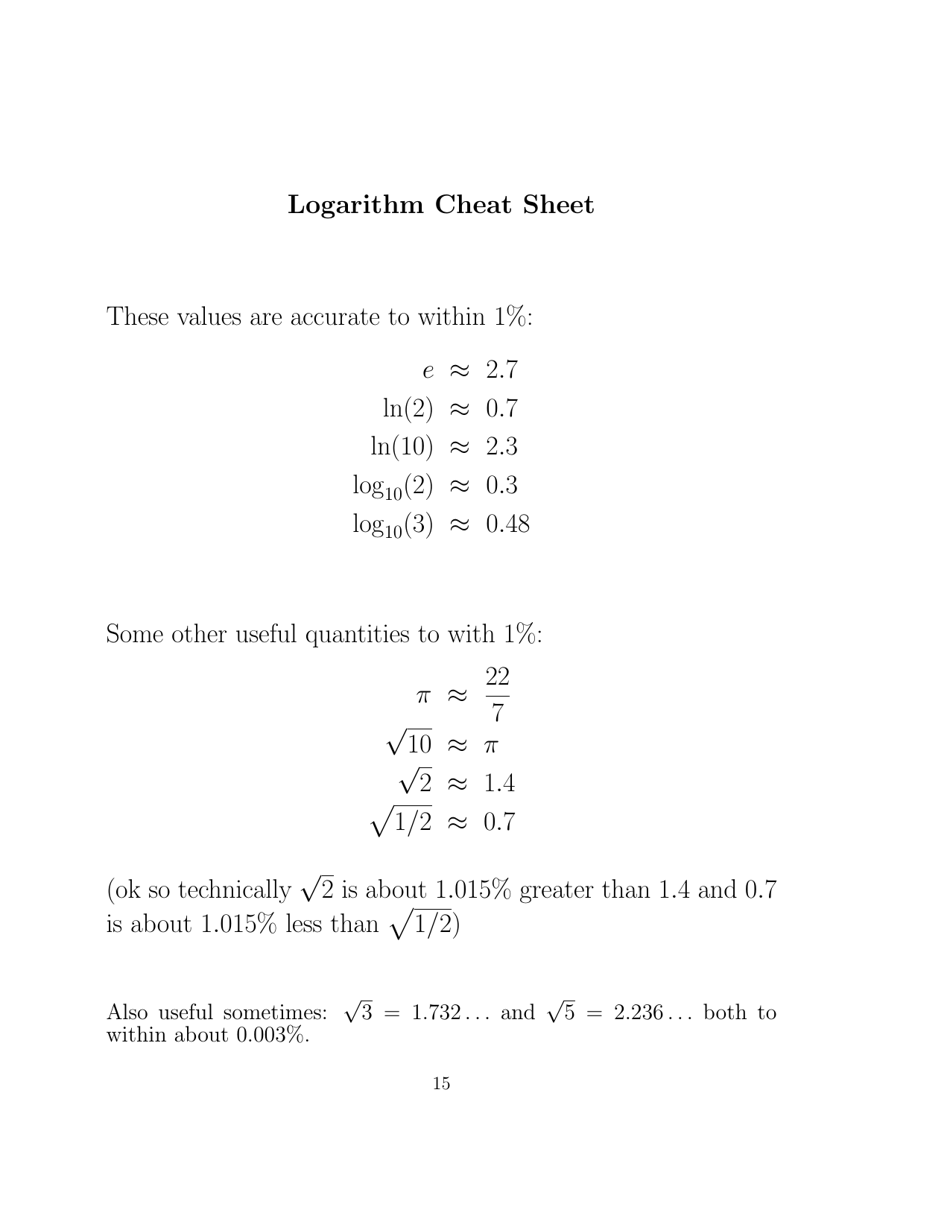
Q: What is the natural logarithm?
A: The natural logarithm is a logarithm with base e, where e is an irrational constant approximately equal to 2.71828.

Q: What are the properties of logarithms?
A: The properties of logarithms include the product rule, quotient rule, and power rule, similar to the properties of exponents.

Q: What is the product rule for logarithms?
A: The product rule states that the logarithm of a product of two numbers is equal to the sum of the logarithms of the individual numbers.

Q: What is the quotient rule for logarithms?
A: The quotient rule states that the logarithm of a quotient of two numbers is equal to the difference of the logarithms of the individual numbers.

Q: What is the power rule for logarithms?
A: The power rule states that the logarithm of a number raised to an exponent is equal to the exponent multiplied by the logarithm of the base.

Q: What are some common logarithmic identities?
A: Some common logarithmic identities include log base b of 1 equals 0, log base b of b equals 1, and log base b of b to the power of x equals x.

Q: What are some common exponential identities?
A: Some common exponential identities include any number raised to the power of 0 equals 1, any number raised to the power of 1 equals itself, and any number raised to the power of a negative exponent equals its reciprocal.