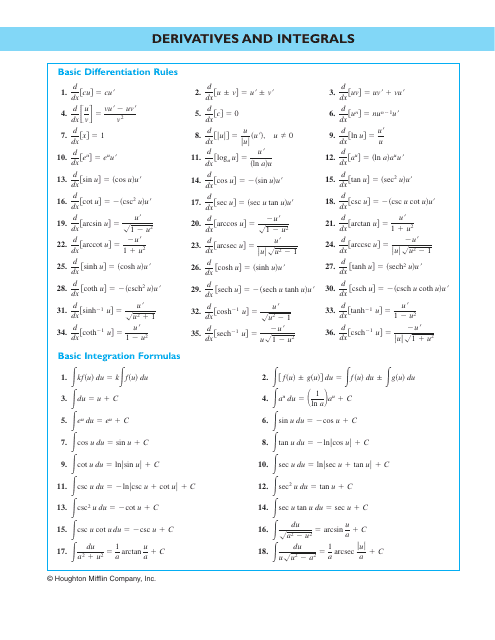
Derivatives and Integrals Cheat Sheet

A derivatives and integrals cheat sheet is a reference guide that provides formulas and techniques for calculating derivatives and integrals in mathematics. It is used to help students and individuals easily understand and apply these concepts in solving mathematical problems.

The derivatives and integrals cheat sheet is typically filed by individual students or professors as a personal study resource. There is no specific entity or organization that formally files it.

FAQ

Q: What is a derivative?A: A derivative measures the rate at which a quantity is changing.

Q: What is an integral?A: An integral is the reverse process of finding the area under a curve.

Q: What are some common derivatives?A: Common derivatives include the derivative of a constant, power rule, product rule, quotient rule, and chain rule.

Q: What are some common integrals?A: Common integrals include the integral of a constant, power rule, exponential function, trigonometric functions, and natural logarithm.

Q: Why are derivatives and integrals important in calculus?A: Derivatives and integrals are fundamental concepts in calculus that help us understand rates of change, find maximums and minimums, and solve various real-world problems.

Q: What are some practical applications of derivatives?A: Derivatives are used in physics to describe the motion of objects, in economics to analyze supply and demand, and in engineering to optimize designs.

Q: What are some practical applications of integrals?A: Integrals are used in physics to calculate displacement, in economics to find total profit or loss, and in engineering to determine the area under complex curves.

Q: Are there any shortcuts or rules for finding derivatives and integrals?A: Yes, there are various rules and formulas that can simplify the process of finding derivatives and integrals, such as the power rule and chain rule for derivatives, and the fundamental theorem of calculus for integrals.

Q: Can derivatives and integrals be used together?A: Yes, derivatives and integrals are closely related and can be used together in calculus to solve problems involving rates of change and accumulation.