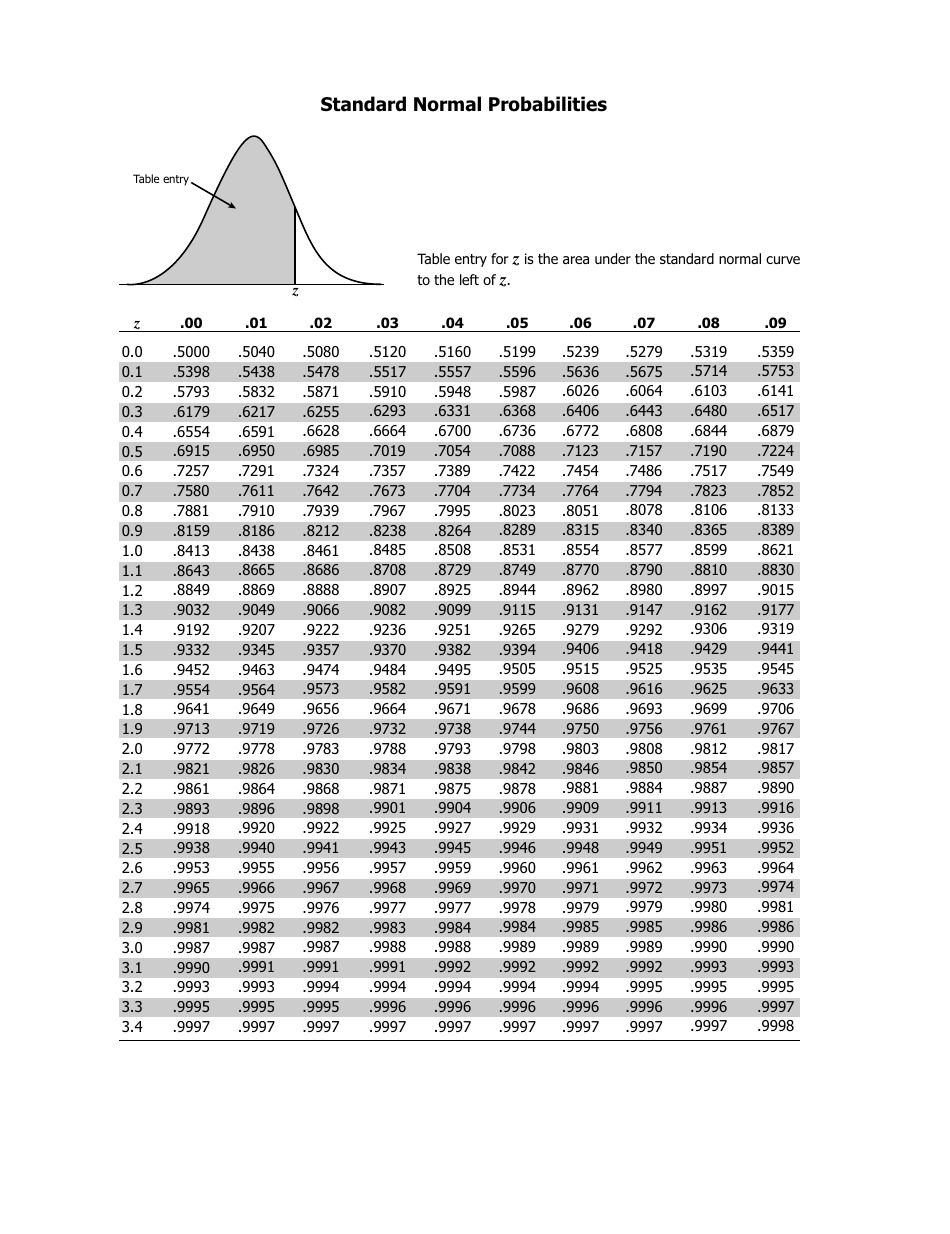
Standard Normal Probabilities - University of Florida

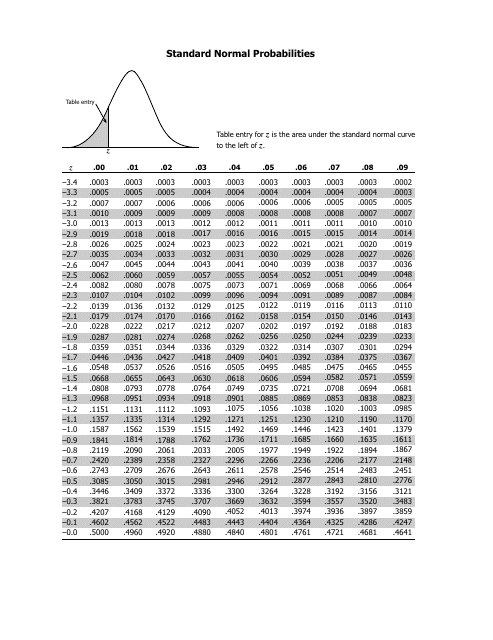
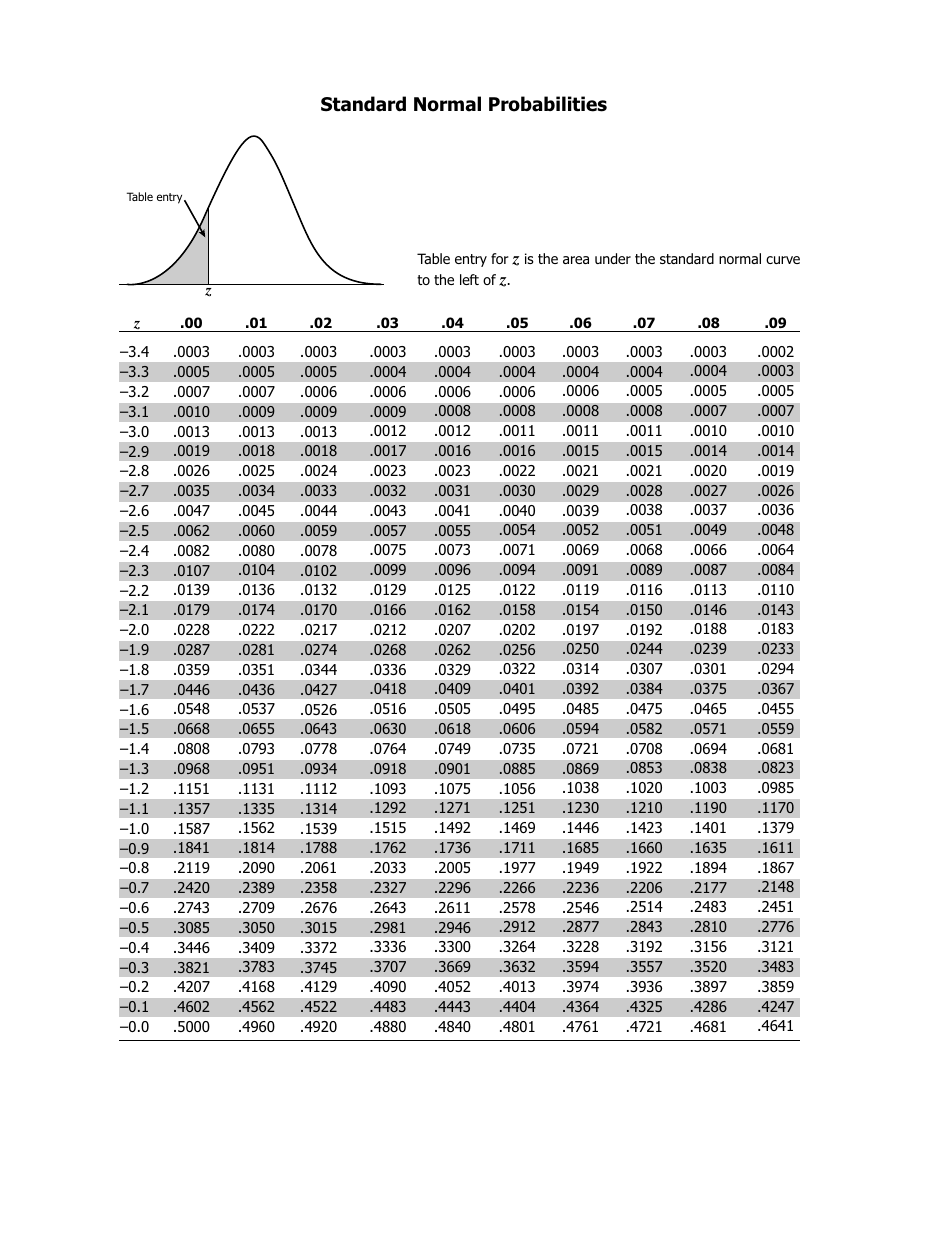
The Standard Normal Probabilities at the University of Florida is a resource that provides information on the probabilities associated with the standard normal distribution. This can be useful in various fields such as statistics, economics, and finance for calculating probabilities and making data-driven decisions.

FAQ

Q: What is a standard normal probability?
A: A standard normal probability refers to the likelihood of a value occurring in a standard normal distribution.

Q: What is a standard normal distribution?
A: A standard normal distribution is a bell-shaped curve with a mean of 0 and a standard deviation of 1.

Q: How are standard normal probabilities calculated?
A: Standard normal probabilities are often calculated using statistical tables or software.

Q: What is the z-score in a standard normal distribution?
A: The z-score is a measure of how many standard deviations a value is away from the mean in a standard normal distribution.

Q: How can I interpret standard normal probabilities?
A: Standard normal probabilities can be interpreted as the likelihood of a value occurring relative to the mean and standard deviation of a standard normal distribution.

Q: Are standard normal probabilities only applicable to a specific context?
A: No, standard normal probabilities can be used in various contexts as long as the data follows a normal distribution or can be transformed into a standard normal distribution.